Division is one of the basic mathematical operations. Basically, division is the ratio between two numbers. The question is how do we perform division operation for two natural numbers? Imagine you have  chocolates and you need to distribute those chocolates to your friends. At that moment, you have
chocolates and you need to distribute those chocolates to your friends. At that moment, you have  friends who are eager to get chocolates from you. How you are going to distribute those chocolates equally to your friends? By divide
friends who are eager to get chocolates from you. How you are going to distribute those chocolates equally to your friends? By divide  by
by  and, in mathematical language, this is how you need to write:
and, in mathematical language, this is how you need to write:
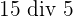 or
or 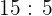 or
or 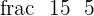
All the above syntaxes are correct and if you see any of the syntaxes above, it means you need to perform the division operation. When you are dividing, basically you are trying to split the first number into equal parts and the number of equal parts is decided by the second number. In the above example, you need to split  into
into  equal parts. If you give
equal parts. If you give  chocolates to each of your friends, that means you equally divide
chocolates to each of your friends, that means you equally divide  chocolates within your friends, hence:
chocolates within your friends, hence:

Where,
 is the dividend,
is the dividend,
 is the divisor,
is the divisor,
and  is the quotient.
is the quotient.

Types of Divisions
The above division might look easy but things get complicated as you move forward and that is why you should be aware of types of divisions. There are two types of division that you might encounter.
1. Exact Division
If you divide a number and the answer that you receive is zero that means it is an exact division. An exact division will always have the remainder zero.
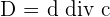
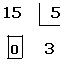
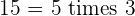
2. Not Exact Division
If you divide a number but in the end, you still get a number which isn't divisible by the divisor, that is the case of not exact division. The number which isn't divisible by the divisor is called the remainder. In simple words, a division is not exact when the remainder is not zero.
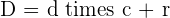
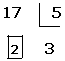
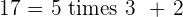
Properties of the Division of Natural Numbers
Like every mathematical operation, division of natural numbers also has some properties. Below are all the properties:
Property No.1: Doesn't Follow Closure Property
This property tells that if you divide two natural numbers this will result in a new number which will not always be a natural number.

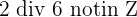
Property No.2: It is not Commutative
When you are dividing two natural numbers, the order does change the division.
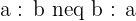
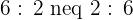
Property No.3: Zero Dividend
If you divide zero by any number, the result will always be equal to zero.
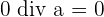
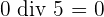
Property No.4: Zero Divisor
Any number divided by zero will always result in infinity.












I’m afraid this article (on cardinal numbers) has an unfortunate error. Zero must be considered a cardinal number. The definition of a cardinal number given by the Penguin Dictionary of Mathematics is “A number that indicates the number of elements in a set.” It also defines a null set or empty set containing no elements. The cardinality of this set is 0.