Chapters
Functions are tested with different domains to find their range. For example, you are asked to find the range of function, 
. When you insert domain values, you get a definite answer. When you place the value zero, it results in infinity which is undefined. Now, you will try putting values near zero such as 
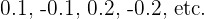to check whether you are still getting infinity. These values do give a definite answer, it means that at zero, the function becomes undefined. In the language of limits, the function approaches infinity when you input zero in the function. The purpose of the limit is to test the value whether it gives you infinity or not.
Limit of a Function at a Point
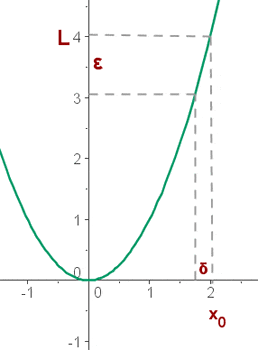
When you apply the limit to a function, it approaches that point. Normally, it approaches the point from a positive direction to that point but you can change the direction of the approaching. For example, limit is applied on function,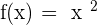 
at 
. It will approach from positive direction to and below are the results:
| x | f(x) |
|---|---|
| 1,9 | 3,61 |
| 1,99 | 3,9601 |
| 1,999 | 3,996001 |
| ... | ... |
| ↓ | ↓ |
| 2 | 4 |
As you can check the result that the output of the function is converging to 
. Did you notice something? All the values approaching are in 
s. Let's say you want to test the continuity of the function, you need to check whether you are still getting from the opposite direction? It means that the limit will be approached from the negative direction to that point. Below are the results:
| x | f(x) |
|---|---|
| 2,1 | 4.41 |
| 2,01 | 4,0401 |
| 2,001 | 4,004001 |
| ... | ... |
| ↓ | ↓ |
| 2 | 4 |
Since the limit is coming from the negative direction, that means that all the value will be greater than but they will be close to like in the above table, 
.
In nutshell, the limit of the function, f(x), at point, 
, is essentially the value of y when x approaches x0. In the example, when x becomes closer to 2 from the left and right side the value of the function will approach 4.
It is said that the limit of the function, f(x), as x tends to x0, is L. If a real positive number is set, ε, greater than zero, there will be a positive number, δ, depending on ε, for all the values of x that differ from x0 that fulfil the condition |x - x0| < δ, and holds that |f(x) - L| <ε.
Did you like this article? Rate it!
Loading...
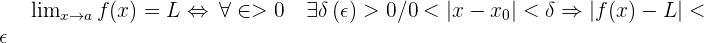
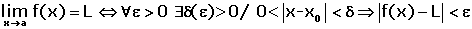
Thank you
Thank you Abbas! Good luck with your studies!
With regard to the Zero Over a Number item, is there a mis-statement? It’s immediately followed by “If a number is divided by zero which means that the numerator is zero and the denominator is the number, then the result is zero.”
Hi Mark,
You’re absolutely right to raise the question — there does appear to be a misstatement in that sentence. The phrase “If a number is divided by zero, which means that the numerator is zero and the denominator is the number…” is indeed misleading and should be corrected.
To clarify:
Zero divided by a number (e.g. 0 ÷ 5) equals 0.
A number divided by zero (e.g. 5 ÷ 0) is undefined.
We’ll update the sentence to reflect the correct mathematical explanation. We appreciate you catching that and helping us improve the accuracy of the content!
There is more than one size of infinity, though. What if you multiply the infinity of the whole numbers (Aleph-0) by the infinity of the real numbers (fraktur-c)?
Thanks a lot to you for this essentiol article.
Hi Piyash! Thanks for your comment, great to hear that you found this useful!
Very nice
🚇🌋⚓️🛳️✈️🛸🚅🛬🚆♟️